Polyiamonds

Figure 1

Link to post on Polyominoes from March 1st 2020.

Just recently I turned 26166 days old and one of the properties of this number is that it is a member of OEIS A000577: number of polyiamonds with \(n\) cells (turning over allowed, holes allowed, must be connected along edges). In the case of 26166, the value of \(n\) is 14. Figure 1 shows a polyiamond with 13 cells that conforms with the sequence's restrictions. It has a hole but if that hole were filled by a triangle then there would be 14 triangles and its shape would account for one of the 26166 possible polyiamonds.  

Wolfram Mathworld gives the following definition of a polyiamond:

A generalisation of the polyominoes using a collection of equal-sized equilateral triangles (instead of squares) arranged with coincident sides. Polyiamonds are sometimes simply known as iamonds.

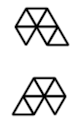
Figure 2 shows the possible polyiamond shapes formed by one up to six polyiamonds. The categories can be identified by a prefix \(n\) indicating the number of polyiamonds that comprise them viz. \(n\)-polyiamonds. So Figure 2 shows the different types of 1-polyiamonds up to 6-polyiamonds. Alternatively, a 6-polyiamond can be referred to as a hexiamond and so forth as shown in the diagram.

Figure 2

Figure 3

Generally, mirror images are not considered distinct. In other words, any polyiamond can be picked up, flipped over and the two mirror images are considered the same. See Figure 3. Once there are nine or more polyiamonds, holes are possible. Some of these are shown in Figure 4. So returning to OEIS A000577 and Figure 2, it can be seen why the initial terms are 1, 1, 1, 3, 4 and 12. The full sequence, up to \(n=14\) is 1, 1, 1, 3, 4, 12, 24, 66, 160, 448, 1186, 3334, 9235, 26166 and 73983. The terms getting rapidly larger as \(n\) increases. 

The hexiamonds are given special names. Again, quoting from Wolfram Mathworld and referring to Figure 2, these are:

Top row: bar, crook, crown, sphinx, snake, and yacht.

Bottom row: chevron, signpost, lobster, hook, hexagon, butterfly.

Polyiamonds with holes are shown in Figure 4. The number of holes associated with the different categories of polyiamonds are given by OEIS A070764: number of polyiamonds with \(n\) cells, with holes. The sequence, up to \(n\)=17, runs: 0, 0, 0, 0, 0, 0, 0, 0, 1, 4, 25, 108, 450, 1713, 6267, 21988, 75185.

Figure 4

These shapes can be put together in interesting ways. For example, Figure 5 shows how 9 hexiamonds can be assembled to form a hexagon while Figure 6 shows how 19 hexiamonds can be assembled to form a more complex hexagonal shape:

Figure 5: source

Figure 6: source

Lots of interesting information, puzzles, tables etc. to be found at:

• http://puzzler.sourceforge.net/
• http://www.recmath.com/PolyPages/PolyPages/index.htm?Polyiamonds.htm
• https://abarothsworld.com/Puzzles/Polyiamonds/Polyiamonds.htm
• https://polyform.fandom.com/wiki/Polyiamond
• http://www.mathpuzzle.com/iamond.htm

Of course, another approach is to look at the 3-D connections of polyiamonds. Figure 7 shows how a 20-polyiamond folds to form an icosahedron.

Figure 7: source

This leads into the "deltahedron (plural deltahedra), a polyhedron whose faces are all equilateral triangles. The name is taken from the Greek upper case delta (Δ), which has the shape of an equilateral triangle. There are infinitely many deltahedra, but of these only eight are convex, having 4, 6, 8, 10, 12, 14, 16 and 20 faces". Source.

Clearly, there's a lot of room for exploration in this topic but that's enough for this post.