26562: A Mid-Millennial Palindrome

Every one hundred days, as I track my diurnal age, a palindromic numbered day comes my way. Today is day 26562. Sometimes, like today's number, I create a post dedicated to the palindrome. I didn't do this for 26462 but prior to that I've posted about:

• 26362: Another Special Palindrome on June 6th 2021
• 26262: A Special Palindrome on February 26th 2021
• Palindromic Cyclops Numbers (in particular 26062) on August 10th 2020

In the transition from one millennium to another, the gap increases to 110 days e.g. 25952 to 26062. Other posts relating to palindromes include:

• L-th Order Palindromes on January 24th 2019
• Lycrel Numbers on September 14th 2016
• Remembering Reverse and Add, Palindromes and Trajectories on June 22nd 2016
• 22, Reverse and Add on January 7th 2016

Of course, my next palindromic day 26662 will be spectacular but today I'm focused on the less spectacular 26562. Here are some of its properties:

PROPERTY ONE

A046263

Largest palindromic substring in \(5^n\).

26562 makes regular appearances in OEIS A046263, in fact it appears in every 16th term:

1, 5, 5, 5, 6, 5, 6, 8, 9, 9, 656, 828, 414, 22, 515, 757, 878, 939, 26562, 9, 9, 8, 101, 55, 464, 3223, 11611, 969, 252, 626, 515, 656, 696, 44, 26562, 7337, 51915, 75957, 797, 989, 949, 747, 787, 9739379, 86968, 707, 4224, 1001, 929, 646, 26562, 61616, 63336, ...

Here are the powers of \(n\) in which it appears up to 100:

• \(5^{18}\) = 3814697265625
• \(5^{34}\) = 582076609134674072265625
• \(5^{50}\) = 88817841970012523233890533447265625
• \(5^{66}\) = 13552527156068805425093160010874271392822265625
• \(5^{82}\) = 2067951531382569187178521730174907133914530277252197265625
• \(5^{98}\) = 315544362088404722164691426113114491869282574043609201908111572265625

PROPERTY TWO

A046394

Palindromes with exactly 4 distinct prime factors.

Here are the initial members and their factorisations:

  Palindrome   Factorisation

  858          2 * 3 * 11 * 13

  2002         2 * 7 * 11 * 13

  2442         2 * 3 * 11 * 37

  3003         3 * 7 * 11 * 13

  4774         2 * 7 * 11 * 31

  5005         5 * 7 * 11 * 13

  5115         3 * 5 * 11 * 31

  6666         2 * 3 * 11 * 101

  10101        3 * 7 * 13 * 37

  15351        3 * 7 * 17 * 43

  17871        3 * 7 * 23 * 37

  22422        2 * 3 * 37 * 101

  22722        2 * 3 * 7 * 541

  24242        2 * 17 * 23 * 31

  26562        2 * 3 * 19 * 233

  26962        2 * 13 * 17 * 61

  28482        2 * 3 * 47 * 101

  35853        3 * 17 * 19 * 37

  36363        3 * 17 * 23 * 31

PROPERTY THREE

A045960

Palindromic even lucky numbers.

The initial members are:

2, 4, 6, 22, 44, 212, 262, 282, 434, 474, 646, 666, 818, 838, 868, 2442, 2662, 2772, 4884, 4994, 6666, 6886, 8118, 8338, 20202, 20402, 21012, 21812, 22322, 22422, 22922, 23332, 23532, 24042, 25652, 26162, 26262, 26562, 26762, 27372, 28682, 40204, 40804

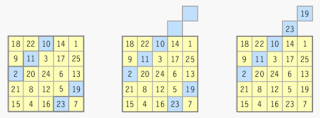
Figure 1 reminds us what even lucky numbers are:

Figure 1: source

PROPERTY FOUR

A317976

a(n) = 2(a(n-1)+a(n-2)+a(n-3))-a(n-4) for n >= 4, with initial terms 0,0,1,0.

The terms quickly increase in size and the initial terms are:

0, 0, 1, 0, 2, 6, 15, 46, 132, 380, 1101, 3180, 9190, 26562, 76763, 221850, 641160, 1852984, 5355225, 15476888, 44729034, 129269310, 373595239, 1079710278, 3120420620, 9018182964, 26063032485, 75323561860, 217689133998, 629133273722, 1818228906675, 5254779066930, 15186593360656, 43890069394800, 126844654738097

The generating function for these terms is:$$ \frac{x^2(1 - 2x) }{1 - 2x - 2x^2 - 2x^3 + x^4}$$PROPERTY FIVE

A261924

Numbers that are the sum of two palindromes of the same length.

In the case of 26562, there are 21 such palindromic pairs:

• (16561, 10001)
• (16461, 10101)
• (16361, 10201)
• (16261, 10301)
• (16161, 10401)
• (16061, 10501)
• (15551, 11011)
• (15451, 11111)
• (15351, 11211)
• (15251, 11311)
• (15151, 11411)
• (15051, 11511)
• (14541, 12021)
• (14441, 12121)
• (14341, 12221)
• (14241, 12321)
• (14141, 12421)
• (14041, 12521)
• (13531, 13031)
• (13431, 13131)
• (13331, 13231)

The pair (13431, 13131) is of particular interest because its members share no digits in common with their addend 26562.

So the wait is on now for my next palindromic day, 26662, which interestingly falls on Saturday, April 2nd 2022, the day before my 73rd birthday. However, my 73rd Solar Return  occurs at 8:34pm on April 2nd. My birthday will thus occur on a Sunday just as on the day I was born. The 666 sequence of numbers will span ten days:

26660, 26661, 26662, 26663 (birthday), 26664, 26665, 26666, 26667, 26668, 26669

While on the subject of palindromes, I came across a tweet that I'd posted on a very special day. See Figure 2. The date was Thursday, February 4th 2010, almost 12 years ago. It's hard to read but on that date I was 22,222 days old.

Figure 2

Mathematical Properties of 2022

It's always interesting to look at the mathematical properties of the number being used to mark the year ahead in the Anno Domini or AD system. At the time of creation of this post, that number is 2022. First and foremost, its factors should be considered and these are 2, 3 and 337 marking it as a so-called sphenic number because it is the product of three distinct primes. 

I've written about these sorts of numbers in two posts titled Sphenic Numbers on June 25th 2018 and Sphenic Numbers Revisited on January 1st 2018. All sphenic numbers have exactly eight divisors and in the case of 2022, these are 1, 2, 3, 6, 337, 674, 1011 and 2022.

2022 has the distinction of belonging to OEIS A105936:

A105936

Numbers that are the product of exactly 3 primes and are of the form prime(\(n\)) + prime(\(n\)+1).

The initial members are:

8, 12, 18, 30, 42, 52, 68, 78, 138, 172, 186, 222, 258, 268, 410, 434, 508, 548, 618, 668, 762, 772, 786, 892, 906, 946, 978, 1002, 1030, 1132, 1334, 1374, 1446, 1542, 1606, 1758, 1866, 1878, 1948, 2006, 2022, 2252, 2334, 2414, 2452, 2468, 2486, 2572, 2588

It should be noted that not all members of this sequence are sphenic. For example, 12 is a member but it is not a product of three distinct primes because the factor 2 is repeated. In the case of 12, it can be seen that it is the sum of two consecutive primes viz. 5 and 7. For 2022, the two consecutive primes are 1009 and 1013. The fact that they are separated by 4 makes them cousin primes.

Consulting the Online Encyclopaedia of Integer Sequences or OEIS, the second sequence of interest is OEIS A141769:

A141769

Beginning of a run of 4 consecutive Niven (or Harshad) numbers.

The initial members of the sequence are:

1, 2, 3, 4, 5, 6, 7, 510, 1014, 2022, 3030, 10307, 12102, 12255, 13110, 60398, 61215, 93040, 100302, 101310, 110175, 122415, 127533, 131052, 131053, 196447, 201102, 202110, 220335, 223167, 245725, 255045, 280824, 306015, 311232, 318800, 325600, 372112, 455422

Harshad or Niven numbers as they are also called are simply numbers that are divisible by their sum of digits. In the case of 2022, it can be seen that it and the three consecutive numbers following it are Harshad. Let's confirm that:$$ \begin{align} \frac{2022}{6}&=337\\ \frac{2023}{7}&=289\\ \frac{2024}{8}&=278\\ \frac{2025}{5}&=405 \end{align}$$ As can be seen such runs are not common. However, it is possible to have runs of up to twenty consecutive Harshad numbers. See Figure 1.

I've written about Harshad numbers in posts titled Harshad Numbers on February 11th 2017 and Harshad Numbers Revisited on June 30th 2018. Figure 1 shows the start of consecutive runs up to 13. Note that the numbers from 1 to 10 are trivially Harshad.

Figure 1: permalink for calculating runs

The next interesting property of 2022 is that not only is it a Harshad number but so are all its powers up to the 7th power. Figure 2 confirms this (SOD stands for Sum Of Digits):

Figure 2: permalink

This property constitutes OEIS A135192:

A135192

Numbers \(n\) that raised to the powers from 1 to \(k\) (with \(k \geq 1 \)) are multiple of the sum of their digits (\(n\) raised to \(k\)+1 must not be a multiple). Case \(k\)=7.

The initial members of the sequence are:

126, 480, 660, 810, 882, 1020, 1134, 1170, 1260, 1320, 1560, 1590, 2022, 3042, 3222, 4662, 4800, 5670, 5940, 6240, 6600, 7110, 7452, 8100, 8442, 8550, 8820, 8880, 9510, 10110, 10200, 10350, 10620, 10890, 11010, 11106, 11130, 11340, 11460, 11700, 11970

Not only is 2022 a Harshad number but it is also an admirable number, the latter being defined as a number whose sum of proper divisors is equal to the number itself with the proviso that one of the divisors is negative. In the case of 2022, its proper divisors are 1, 2, 3, 6, 337, 674 and 1011 which sum to 2034. However, if the +6 is made -6, then the sum becomes 2022. Moreover, 6 happens to be the digit sum of 2022 since 2 + 2 + 0 + 2 =6. This qualifies 2022 for membership is OEIS A111948: 

A111948

Admirable Harshad numbers \(n\) such that the subtracted divisor is equal to the digital sum of \(n\).

The initial members of the sequence are:

24, 42, 114, 222, 402, 2022, 2202, 7588, 8596, 10014, 11202, 12102, 17668, 21102, 27748, 29764, 31002, 32788, 39844, 42868, 43876, 45388, 46396, 48916, 49924, 55972, 56476, 57484, 58492, 65548, 66556, 69076, 70588, 71596, 78148, 81676

2022 is also a self number because there is no number that, when added to its sum of digits, produces 2022. Thus it both a Harshad and a self number which qualifies it for membership in OEIS  A003219:

A003219

Self numbers divisible by sum of their digits (or, self numbers which are also Harshad numbers).

The initial terms of the sequence are:

1, 3, 5, 7, 9, 20, 42, 108, 110, 132, 198, 209, 222, 266, 288, 312, 378, 400, 468, 512, 558, 648, 738, 782, 804, 828, 918, 1032, 1098, 1122, 1188, 1212, 1278, 1300, 1368, 1458, 1526, 1548, 1638, 1704, 1728, 1818, 1974, 2007, 2022, 2088, 2112, 2156, 2178 

I've written about self numbers in a post titled Self Numbers and Junction Numbers on October 25th 2018.

The next two interesting properties of 2022 involve primes (as did OEIS A105936 mentioned earlier). The first property qualifies it for admission in OEIS A023523 (permalink):

A023523

a(\(n\)) = prime(\(n\))*prime(\(n\)-1) + 1.

The initial members of the sequence are with prime(0) being considered as 1:

3, 7, 16, 36, 78, 144, 222, 324, 438, 668, 900, 1148, 1518, 1764, 2022, 2492, 3128, 3600, 4088, 4758, 5184, 5768, 6558, 7388, 8634, 9798, 10404, 11022, 11664, 12318, 14352, 16638, 17948, 19044, 20712, 22500, 23708, 25592, 27222, 28892

In the case of 2022, it is the product of the 14th prime (43) and the 15th prime (47) plus 1.

The second interesting property of 2022 involving primes qualifies it for membership in OEIS A064403:

A064403

Numbers \(k\) such that prime(\(k\)) + \(k\) and prime(\(k\)) - \(k\) are both primes.

The initial members of this sequence are:

4, 6, 18, 42, 66, 144, 282, 384, 408, 450, 522, 564, 618, 672, 720, 732, 744, 828, 858, 1122, 1308, 1374, 1560, 1644, 1698, 1776, 1848, 1920, 2022, 2304, 2412, 2616, 2766, 2778, 2874, 2958, 2970, 3036, 3042, 3240, 3258, 3354, 3360, 3432, 3540, 3594, 3732

In the case of 2022, the two primes are 19603 and 15559 respectively. 

This next property of 2022 is quite unusual and took me some time to fully grasp. This property qualifies the number for membership in OEIS A335600:

A335600

The poor sandwiches sequence.

The sequence runs:

2, 1, 110, 10, 1101, 11010, 3, 330, 30, 3303, 33030, 4, 440, 40, 4404, 44040, 5, 550, 50, 5505, 55050, 6, 660, 60, 6606, 66060, 7, 770, 70, 7707, 77070, 8, 880, 80, 8808, 88080, 9, 990, 90, 9909, 99090, 11, 101, 1010, 22, 20, 202, 220, 2022, 2020, 33, 303, 3030, 44, 404, 4040, 55, 505, 5050, 66, 606, 6060, 77

 The OEIS comments help explain what it's all about:

Imagine we would have a pair of adjacent integers in the sequence like [1951, 2020]. The sandwich would then be made of the rightmost digit of a(n), the leftmost digit of a(n+1) and, in between, the absolute difference of those two digits. The pair [1951, 2020] would then produce the (poor) sandwich 112. 

Why poor? Because a rich sandwich would insert the sum of the digits instead of their absolute difference - that is 132 in this example. Please note that the pair [2020, 1951] would produce the poor and genuine sandwich 011 (we keep the leading zero: these are sandwiches after all, not integers).

Now we want the sequence to be the lexicographically earliest sequence of distinct positive terms such that the successive sandwiches emerging from the sequence rebuild it, digit after digit.

EXAMPLE

The first successive sandwiches are: 211, 101, 011, 011, 101, 033,...

The first one (211) is visible between a(1) = 2 and a(2) = 1; we get the sandwich by inserting the difference 1 between 2 and 1.

The second sandwich (101) is visible between a(2) = 1 and a(3) = 110; we get this sandwich by inserting the difference 0 between 1 and 1.

The third sandwich (011) is visible between a(3) = 110 and a(4) = 10; we get this sandwich by inserting the difference 1 between 0 and 1; etc.

The successive sandwiches rebuild, digit by digit, the starting sequence.

2022 is what is called an untouchable number because it is not equal to the sum of the proper divisors of any number. The untouchable numbers, up to and including 2022, are:

2, 5, 52, 88, 96, 120, 124, 146, 162, 188, 206, 210, 216, 238, 246, 248, 262, 268, 276, 288, 290, 292, 304, 306, 322, 324, 326, 336, 342, 372, 406, 408, 426, 430, 448, 472, 474, 498, 516, 518, 520, 530, 540, 552, 556, 562, 576, 584, 612, 624, 626, 628, 658, 668, 670, 708, 714, 718, 726, 732, 738, 748, 750, 756, 766, 768, 782, 784, 792, 802, 804, 818, 836, 848, 852, 872, 892, 894, 896, 898, 902, 926, 934, 936, 964, 966, 976, 982, 996, 1002, 1028, 1044, 1046, 1060, 1068, 1074, 1078, 1080, 1102, 1116, 1128, 1134, 1146, 1148, 1150, 1160, 1162, 1168, 1180, 1186, 1192, 1200, 1212, 1222, 1236, 1246, 1248, 1254, 1256, 1258, 1266, 1272, 1288, 1296, 1312, 1314, 1316, 1318, 1326, 1332, 1342, 1346, 1348, 1360, 1380, 1388, 1398, 1404, 1406, 1418, 1420, 1422, 1438, 1476, 1506, 1508, 1510, 1522, 1528, 1538, 1542, 1566, 1578, 1588, 1596, 1632, 1642, 1650, 1680, 1682, 1692, 1716, 1718, 1728, 1732, 1746, 1758, 1766, 1774, 1776, 1806, 1816, 1820, 1822, 1830, 1838, 1840, 1842, 1844, 1852, 1860, 1866, 1884, 1888, 1894, 1896, 1920, 1922, 1944, 1956, 1958, 1960, 1962, 1972, 1986, 1992, 2008, 2010, 2022

These numbers constitute OEIS A005114. 

2022 is a primitive abundant number, since it is smaller than the sum of its proper divisors, none of which is abundant.

2022 is a pseudoperfect number, because it is the sum of a subset of its proper divisors which are 1, 2, 3, 6, 337, 674 and 1011. If the subset {337, 674, 1011} is taken then we have 337 + 674 + 1011 = 2020.

2022 is a Zumkeller number, because its divisors can be partitioned in two sets with the same sum (2028). The divisors of 2022 are 1, 2, 3, 6, 337, 674, 1011 and 2022 and these sum to 4056 or 2 x 2028. There are four groupings of two sets satisfying the condition that each sum to 2028. These are:

• 6, 2022 and 1, 2, 3, 337, 674, 1011
• 1, 2, 3, 2022 and 6, 337, 674, 1011
• 6, 337, 674, 1011 and 1, 2, 3, 2022
• 1, 2, 3, 337, 674, 1011 and 6, 2022

There's a lot more that could be said about 2022 but I'll leave off with a reference to "dismal" arithmetic or "lunar" arithmetic as it's apparently been renamed. Here is a link to a PDF file of July 5th 2011 that explains what is meant by dismal arithmetic. It's free to download. The famous N.J.A. Sloane who created the OEIS is a co-author. Here is the abstract:

Dismal arithmetic is just like the arithmetic you learned in school, only simpler: there are no carries, when you add digits you just take the largest, and when you multiply digits you take the smallest. This paper studies basic number theory in this world, including analogues of the primes, number of divisors, sum of divisors, and the partition function.

2022 makes an appearance in lunar arithmetic via OEIS A170806:

A170806

Primes in lunar arithmetic in base 3 written in base 3.

 In Sloane's paper, there is the following definition:

Theorem 9. In base \(b\) dismal arithmetic, \(n\) is prime if and only if the dismal sum of its distinct dismal prime divisors is equal to \(n\).

I won't go further into this arithmetic in this post but perhaps I will later on. 

Bogotá Numbers

Yesterday I turned 26550 days old and one of the properties of 26550 is that it's a Bogotá number. These numbers comprise OEIS A336826:

A336826

Bogotá numbers: numbers k such that k = m*p(m) where p(m) is the digital product of m.

As an example, \(26550 = 295 \times (2 \times 9 \times 5)\). It's interesting to note that the digital product of any number is of the form \(2^a \times 3^b \times 5^c \times 7^c\) where \(a,b,c,d\) are non-negative integers (and thus could include zero values). Numbers of this form are said to be 7-smooth and in general an \(n\)-smooth number is defined as one whose prime factors are all less than or equal to \(n\). In the case of 295 it can be seen that the digital product is of the form \(2^1 \times 3^2 \times 5^1 \times 7^0\).

The initial Bogotá numbers are:

0, 1, 4, 9, 11, 16, 24, 25, 36, 39, 42, 49, 56, 64, 75, 81, 88, 93, 96, 111, 119, 138, 144, 164, 171, 192, 224, 242, 250, 255, 297, 312, 336, 339, 366, 378, 393, 408, 422, 448, 456, 488, 497, 516, 520, 522, 525, 564, 575, 648, 696, 704, 738, 744, 755, 777, 792

In the above list the pairs of consecutive Bogotá numbers are marked in bold. These pairs are not that frequent. Here are the initial pairs (permalink):

  First Member   Second Member

  0              1
  24             25
  2510           2511
  5210           5211
  8991           8992
  56384          56385
  348732         348733
  460719         460720
  867839         867840
  28997919       28997920
  254181375      254181376

Up to one million, the record gaps between successive Bogotá numbers are (permalink):

Number         Next number    Gap

  0              1              1
  1              4              3
  4              9              5
  16             24             8
  25             36             11
  96             111            15
  119            138            19
  144            164            20
  171            192            21
  192            224            32
  255            297            42
  575            648            73
  1778           1872           94
  2688           2784           96
  3942           4092           150
  6125           6288           163
  6792           6966           174
  9144           9333           189
  9468           9666           198
  10820          11034          214
  14256          14488          232
  16119          16408          289
  17295          17676          381
  43040          43512          472
  84996          85608          612
  90272          91152          880
  185616         186672         1056
  213346         214416         1070
  285408         286920         1512
  334950         336672         1722
  853056         855036         1980
  949176         951264         2088

The natural density of Bogotá numbers is 0 (link) and the numbers less than or equal to \(10^n\) with \(n=0,1, 2, \dots, 9\) are 2, 4, 19, 67, 280, 1166, 4777, 19899, 82278, and 340649 respectively (link).

Bogotá of course is the capital of Columbia and these numbers were so named by Tomás Uribe and Juan Pablo Fernández based on similarity to the construction of the Colombian numbers or self numbers. These latter numbers are ones that cannot be formed from \(m\) + digit sum of \(m\) for some positive integer \(m\). I've written about these in a post on October 25th 2018 titled Self Numbers and Junction Numbers.

OEIS A336984 lists numbers that are both Columbian and Bogotá numbers:

A336984

Colombian numbers that are also Bogotá numbers.

The initial members of this sequence are:

1, 9, 42, 64, 75, 255, 312, 378, 525, 648, 738, 1111, 1278, 2224, 2448, 2784, 2817, 3504, 3864, 3875, 4977, 5238, 5495, 5888, 8992, 9712, 10368, 11358, 11817, 12348, 12875, 13136, 13584, 13775, 13832, 13944, 15351, 15384, 15744, 15900, 16912, 17768, 18095, 19344, 20448

Digit Sum Raised to Integer Powers

I'm familiar with what happens when the operation of sum of the digits squared is applied repeatedly to a number. Either the number 1 is reached or the loop {4, 16, 37, 58, 89, 145, 42, 20} is entered. Numbers that reach 1 are called happy numbers and I wrote about these in a blog post on June 26th 2018 titled Happy Numbers.

I hadn't considered what happens when the operation of digit sum squared is repeatedly applied. Let's highlight the difference between the two operations using my diurnal age of 26548 as an example.$$26548 \rightarrow 2^2+6^2+5^2+4^2+8^2=4+36+25+16+64=145\\26548 \rightarrow (2+6+5+4+8)^2=25^2=625$$In the case of the sum of the digits squared, it can be seen that continuing the operation leads to a loop because:$$145 \rightarrow 1^2+4^2+5^2=42$$However, in the case of the square of the digit sum, a loop is also entered because:$$625 \rightarrow (6+2+5)^2 = 169 \rightarrow (1+6+9)^2=256 \rightarrow (2+5+6)^2=169$$Nearly 45% of all numbers will enter this loop. Another 33% will end in 81. For example, 26547 follows this trajectory:$$26547 \rightarrow 576 \rightarrow 324 \rightarrow 81$$Another 22% will end in 1 and, in general, it seems that all numbers will either end in 1 or 81 or enter the loop {169, 256]. Here is a permalink to SageMathCell that will confirm this. The algorithm is easily modifiable to accommodate powers greater than 2 and so what happens if the digit sum is repeatedly raised to the third, fourth, fifth powers etc. can be investigated. Let's look next at the digit sum cubed.

What we find is that almost 32% of numbers enter the loop {6859, 21952}:$$6859 \rightarrow 28^3=21952 \rightarrow 19^3=6859$$All other numbers end in 1, 512, 4913, 5832, 17576 or 19683 with percentages of approximately 1.5%, 7.6%, 13.0%, 25.7%, 12.7% and 7.5% respectively:$$ \begin{align} 1 \rightarrow 1^3 &= 1\\512 \rightarrow 8^3 &= 512\\4913 \rightarrow 17^3 &= 4913\\5832 \rightarrow 18^3 &= 5832\\17576 \rightarrow 26^3 &= 17576\\19683 \rightarrow 27^3 &=19683 \end{align}$$When we raise the digit sum repeatedly to the fourth power, we find that again that some numbers enter the loop {104976, 531441}, about 33% in total:$$104976 \rightarrow 27^4= 531441 \rightarrow 18^4 = 104976$$All other numbers end in 1, 2401, 234256, 390625, 614656 or 1679616 with approximate percentages of 12.1%, 6.0%, 22.2%, 16.2%, 10.1% and 0.7% respectively:$$ \begin{align}1 \rightarrow 1^4 &= 1\\2401 \rightarrow 7^4 &= 2401\\234256 \rightarrow 22^4&= 234256\\ 390625 \rightarrow 25^4&= 390625\\614656 \rightarrow 28^4 &=614656\\ 1679616 \rightarrow 36^4 &=1679616 \end{align}$$When we raise the digit sum repeatedly to the fifth power, about 56% of numbers enter the loops {16807, 5153632, 9765625, 102400000} or {6436343, 20511149} or {28629151, 45435424}. All other numbers end in 1, 17210368, 52521875, 60466176 or 205962976 with approximate percentages of 1.0%, 4.3%, 11.1%, 33.3% and 5.8% respectively:$$ \begin{align}1 \rightarrow 1^5 &= 1\\17210368 \rightarrow 28^5 &= 17210368\\52521875 \rightarrow 35^5 &= 52521875\\60466176 \rightarrow 36^5&= 60466176\\205962976 \rightarrow 46^5 &=205962976\end{align}$$I could go on but the general pattern is clear.

Thue-Morse Constant

On June 20th 2020, I made a post titled Prouhet-Thue-Morse Sequence named for Eugène Prouhet, Axel Thue, and Marston Morse (the Prouhet reference is sometimes omitted). By the way, the Thue part is named after Axel Thue, whose name is pronounced as if it were spelled "Tü" where the ü sound is roughly as in the German word üben. It is incorrect to say "Too-ee" or "Too-eh". Thus sayeth N. J. A. Sloane, June 12th 2018, in his comments about OEIS A010060 that lists the members of the sequence. 

It is a most interesting sequence and my blog post covers it quite well and has links to three interesting YouTube videos. However, there is a so-called Thue-Morse constant that is the topic of this post. The sequence begins:

 0, 1, 1, 0, 1, 0, 0, 1, 1, 0, 0, 1, 0, 1, 1, 0, 1, 0, 0, 1, 0, 1, 1, 0, 0, ... 

If we concatenate these binary digits, we get a binary number:

 \(P=0.0110100110010110100101100..._2\) 

This number can be converted a decimal and is represented by the Greek letter \( \tau \):$$\tau=\sum_{n=0}^{\infty} \frac{t_i}{2^{i+1}}=0.4124540336401075977 \dots$$where \(t_i\) is the \(i^{th}\) element of the binary Thue-Morse sequence. The number has been shown to be transcendental.

Figure 1 provides two interesting expressions for the Thue-Morse constant (source):

Figure 1

I came across the constant by means of my diurnal age investigation, discovering that the number associated with my diurnal age (26547) was a member of OEIS A096394:

A096394

Engel expansion of Thue-Morse constant.

The sequence begins 3, 5, 6, 9, 12, 19, 92, 173, 242, 703, 1861, 3186, 4746, 7843, 26547, ... and the comments state that:$$ 0.4124540336 \dots = \frac{1}{3}+\frac{1}{3 \times 5}+\frac{1}{3 \times 5 \times 6}+\frac{1}{3 \times 5 \times 6 \times 9} + \dots$$I made a post about Engel Expansions way back on September 28th 2016.

If we take 0.412454033640107597783361368258455283089 as an approximation of \(\tau\) and plug this into the SageMathCell formula listed in this post, we do confirm that 26547 is a member. To generate further members of the sequence however, the number of decimal places to which \( \tau \) needs to be approximated must be increased. Here is a permalink to SageMathCell while the code is listed below (blue for input and red for output).

x=0.412454033640107597783361368258455283089

u=x

E=[1]

F=[]

product=1

sum=0

for i in [1..15]:

    a=ceil(1/u)

    u=u*a-1

    E.append(a)

    product=product*a

    sum+=1/product

    F.append(1/product)

print(E, "... this is the Engels expansion")

[1, 3, 5, 6, 9, 12, 19, 92, 173, 242, 703, 1861, 3186, 4746, 7843, 26547] ... this is the Engels expansion

AD and BC Numbers

In my quest to find interesting properties for the successive numbers that constitute my diurnal age, I usually make use of the OEIS and Numbers Aplenty. Occasionally these resources throw up little of interest and, after exhausting additional resources, I'm left to come up with some creative angle of my own.

Today's number, 26541, presented such a challenge and I'm proud to say I rose to the occasion. While professional mathematicians will sneer, recreational mathematical enthusiasts may appreciate my resourcefulness. Here is what I came up with. Decimal numbers when converted to hexadecimal can end in AD and the thought struck me that such numbers would form a sequence. I've entered this sequence into my own private database of sequences. Here it is:

S037: Anno Domini (AD) numbers: decimal numbers that when converted to hexadecimal contain at least three digits satisfying the following criteria: 

• the second last digit is A
• the last digit is D
• all remaining digits are between 0 and 9

Up to 40000, the list of such numbers is as follows:

429, 685, 941, 1197, 1453, 1709, 1965, 2221, 2477, 4269, 4525, 4781, 5037, 5293, 5549, 5805, 6061, 6317, 6573, 8365, 8621, 8877, 9133, 9389, 9645, 9901, 10157, 10413, 10669, 12461, 12717, 12973, 13229, 13485, 13741, 13997, 14253, 14509, 14765, 16557, 16813, 17069, 17325, 17581, 17837, 18093, 18349, 18605, 18861, 20653, 20909, 21165, 21421, 21677, 21933, 22189, 22445, 22701, 22957, 24749, 25005, 25261, 25517, 25773, 26029, 26285, 26541, 26797, 27053, 28845, 29101, 29357, 29613, 29869, 30125, 30381, 30637, 30893, 31149, 32941, 33197, 33453, 33709, 33965, 34221, 34477, 34733, 34989, 35245, 37037, 37293, 37549, 37805, 38061, 38317, 38573, 38829, 39085, 39341

All the numbers differ by 256 except for a regular jump of 1792 or 7 x 256 after every 9th number. In fact, all the numbers equal 173 mod 256 and 173 is the decimal value of AD. The first number in the sequence, 429 = 256 + 173, converts to 1AD while the last number, 39341 = 9 x 4096 + 6 x 256 + 173, converts to 99AD. The number associated with my diurnal age, 26541, converts to 67AD. Here is a permalink to the code that I used in SageMathCell.

The logical extension of course is to identify BC numbers and that is what I've done in another private sequence:

S038: Before Christ (BC) numbers: decimal numbers that when converted to hexadecimal contain at least three digits satisfying the following criteria: 

• the second last digit is B
• the last digit is C
• all remaining digits are between 0 and 9

Up to 40000, the members of this sequence are:

444, 700, 956, 1212, 1468, 1724, 1980, 2236, 2492, 4284, 4540, 4796, 5052, 5308, 5564, 5820, 6076, 6332, 6588, 8380, 8636, 8892, 9148, 9404, 9660, 9916, 10172, 10428, 10684, 12476, 12732, 12988, 13244, 13500, 13756, 14012, 14268, 14524, 14780, 16572, 16828, 17084, 17340, 17596, 17852, 18108, 18364, 18620, 18876, 20668, 20924, 21180, 21436, 21692, 21948, 22204, 22460, 22716, 22972, 24764, 25020, 25276, 25532, 25788, 26044, 26300, 26556, 26812, 27068, 28860, 29116, 29372, 29628, 29884, 30140, 30396, 30652, 30908, 31164, 32956, 33212, 33468, 33724, 33980, 34236, 34492, 34748, 35004, 35260, 37052, 37308, 37564, 37820, 38076, 38332, 38588, 38844, 39100, 39356

Again, all the numbers differ by 256 except for a regular jump of 1792 or 7 x 256 after every 9th number. Here, all the numbers equal 188 mod 256 and 188 is the decimal value of BC. The first number in the sequence, 444 = 256 + 188, converts to 1BC while the last number, 39356 = 9 x 4096 + 9 x 256 + 188, converts to 99BC. Here is a permalink to the code that I used in SageMathCell. 

I know that BCE and CE can be used instead of BC and AD but I refuse to use this system. Anyone so inclined however, could modify my existing algorithms or create algorithms of their own in order to generate hexadecimal numbers ending in BCE and CE.

The numbers don't have to be necessarily hexadecimal because the letters used in my system are A, B, C and D and thus will work for bases 14 and 15 and 17 up to 36. I just chose hexadecimal because of its widespread use in technology. 

It's interesting that 67 AD has associations with Christianity because this year marked the end of St. Paul's journeying around the Mediterranean. See Figure 1.

Figure 1: source

This post marks my 100th post for the year 2021, an annual record that I've never even approached before. Previously, my annual totals were:

• 2020: 68 posts
• 2019: 56 posts
• 2018: 68 posts
• 2017: 36 posts
• 2016: 42 posts
• 2015: 12 posts

I started this blog in September of 2015, shortly after my retirement from teaching.

Unremarkable Numbers

Every now and again, as I track my diurnal age, I come across a number that is, for want of a better word, unremarkable. 26534 appears to be such a number. It has two entries in the OEIS and both of them are of the inscrutable variety. Searches elsewhere reveal nothing meaningful either. 

Such apparent "duds" can serve as a stimulus to really look at the number more closely in an effort to extract some properties of interest. In the case of 26534, two properties came to light when I did this. These are:

• each of its five digits is unique (2, 6, 5, 3, 4)
• the digits when ordered are sequential (2, 3, 4, 5, 6)

This becomes important when tracking my diurnal age because, in the span of 10000 numbers from 20000 to 29999, there are very few numbers with this property. There are 120 permutations of the five digits (2, 3, 4, 5, 6) but if we fix the 2 in place then there are only 24 numbers that lie in this range from 20000 to 29999. These are:

23456, 23465, 23546, 23564, 23645, 23654, 24356, 24365, 24536, 24563, 24635, 24653, 25346, 25364, 25436, 25463, 25634, 25643, 26345, 26354, 26435, 26453, 26534, 26543

As can be seen, 26534 is the penultimate number when such numbers are ordered and the final number, 26543, is only nine days away. Another group of 24 numbers can be found for the range from 20000 to 29999 using the digits 1, 2, 3, 4, 5 by fixing the 2 in first place. The numbers in this group are:

21345, 21354, 21435, 21453, 21534, 21543, 23145, 23154, 23415, 23451, 23514, 23541, 24135, 24153, 24315, 24351, 24513, 24531, 25134, 25143, 25314, 25341, 25413, 25431

Using the digits from 0 to 4 and again fixing the 2 in first place, we get another 24 digits in the range from 20000 to 29999. These are:

20134, 20143, 20314, 20341, 20413, 20431, 21034, 21043, 21304, 21340, 21403, 21430, 23014, 23041, 23104, 23140, 23401, 23410, 24013, 24031, 24103, 24130, 24301, 24310

Altogether then there are 72 numbers in the range from 20000 to 29999 that meet the criteria:

• each digit in unique
• the digits when ordered are sequential

Thus, out of 10000 numbers, only 0.72% satisfy the criteria. It turns out that 26534 is rather special after all. Here is a permalink that can be used to generate these numbers.

Star Numbers Revisited

 So called Star Numbers are few and far between. Up to 40,000, they are:

1, 13, 37, 73, 121, 181, 253, 337, 433, 541, 661, 793, 937, 1093, 1261, 1441, 1633, 1837, 2053, 2281, 2521, 2773, 3037, 3313, 3601, 3901, 4213, 4537, 4873, 5221, 5581, 5953, 6337, 6733, 7141, 7561, 7993, 8437, 8893, 9361, 9841, 10333, 10837, 11353, 11881, 12421, 12973, 13537, 14113, 14701, 15301, 15913, 16537, 17173, 17821, 18481, 19153, 19837, 20533, 21241, 21961, 22693, 23437, 24193, 24961, 25741, 26533, 27337, 28153, 28981, 29821, 30673, 31537, 32413, 33301, 34201, 35113, 36037, 36973, 37921, 38881, 39853

Today I happened to turn 26533 days old which is why my attention was drawn to them again. I had posted about these numbers in an eponymous blog post on 7th June 2019. On that occasion I was writing about the star number 25741 that was 109 days away at that point. I was surprised to see that this post came up in fourth position when the phrase "star numbers" was entered into the Google search bar (see Figure 1):

Figure 1

It also attracted two comments that I only just noticed (see Figure 2). I'm reminded that I should check my various blogs for comments on a regular basis, something that I've quite neglected.

Figure 2

In this post I want to look at some interesting properties of this sequence that I didn't cover in that earlier post. The first involves a result for the sum to infinity of the reciprocals of the star numbers:$$ \sum_{n=1}^{\infty} \frac{1}{S_n}=\frac{\pi \tan{\dfrac{\pi}{2 \sqrt{3}}}}{2 \sqrt{3}} \approx 1.15917331963217$$The second infinite sum involves the factorial function:$$ \sum_{n=0}^{\infty} \frac{S_n}{n!}=7 e$$The third infinite sum involves powers of 2:$$ \sum_{n=1}^{\infty} \frac{S_n}{2^n}=25$$These results are listed in Numbers Aplenty but no proofs are supplied, so I'm just listing them here. Wolfram MathWorld supplies a generating function for the star numbers:

It also provides a linear recurrence relation:  

The star numbers form OEIS :

A003154

Centered 12-gonal (dodecagonal) numbers. Also star numbers: 6*n*(n-1) + 1.

In the comments to this sequence in the OEIS, the diagram shown in Figure 3 appears:

Figure 3

So that's about it. In summary, this post is simply meant to supplement my earlier post and I would encourage anyone reading this post to read that as well, because it contains many other interesting facts about star numbers.

Numbers As Sums Of Palindromes

I've written about palindromes before in a variety of posts (just type palindromes into the search box for this site) but thus far I've not mentioned the representation of numbers as a sum of palindromes. Figure 1 shows a screenshot of a tweet from Cliff Pickover's Twitter feed:

Figure 1

I came across this tweet not long ago and immediately wrote a program (permalink) in SageMathCell to determine what these palindromic sums were for any given number. Below is the calculation box.

It turns out that for 26532, my diurnal age today, there are 193 different way to represent it as a sum of three palindromes. Here are examples of just a few of them:

• 11 + 969 +25552
• 414 + 7337 + 18781
• 979 + 1111 + 24442
• 5885 + 6006 + 14641

Most numbers, about 92%, can in fact be represented as a sum of two palindromes in one or more ways. However, 26532 is amongst the 8% minority that cannot. The numbers on either side of it however, can be represented as a sum of two palindromes. The program above can be easily modified to find these palindromes, just replace C = Combinations(P, 3) with C = Combinations(P, 2). Here are the results:

• 26531 = 979 + 25552
• 26533 = 171 + 26362 = 1991 + 24542

Between 26500 and 27000, the numbers that cannot be represented as a sum of two palindromes are:

26502, 26512, 26522, 26532, 26542, 26572, 26582, 26592, 26602, 26612, 26622, 26632, 26642, 26672, 26682, 26692, 26702, 26712, 26722, 26732, 26742, 26772, 26782, 26792, 26802, 26812, 26822, 26832, 26842, 26872, 26882, 26892, 26902, 26912, 26922, 26932, 26942, 26952, 26972, 26982, 26992

There are 41 such numbers representing 8.20% of the total of 500 numbers. It can be noted that all the numbers in this range are even and all end in 2. This pattern seems to repeat for other ranges. 

That's about it, a simple yet interesting property that divides numbers into two categories: those that can be represented as a sum of two palindromes and those that cannot. 

888

PHYSICS

Figure 1: neutron decays into proton, electron and neutrino

Triple 8 is a most interesting number for a variety of reasons. However, today I stumbled upon the number is an unexpected context. In an article in UNIVERSE TODAY dated 11th November 2021, it's stated that:

Together with protons, neutrons make up the nuclei of the atoms we see around us. Within an atomic nucleus, neutrons can be extremely stable. But when a neutron is on its own, it typically decays in a matter of minutes. 

There are a couple of ways we can measure neutron half-life, such as measuring a beam of neutrons or cooling them down and trapping them in a magnetic bottle, but these different methods give different results for the half-life. The methods should give the same result, but they don’t. The beam method gives a lifetime of 888 seconds, while the bottle method gives 879 seconds.

Perhaps there is some systematic error in the methods, but this discrepancy is a problem for fundamental physics. But a new study has measured neutron decay in a third way, by using a spacecraft orbiting the Moon.

The airless surface of the moon is constantly bombarded by cosmic rays. Sometimes a cosmic ray will kick a neutron off the lunar surface. As the neutron speeds away from the Moon, it has a chance of decaying. So the team used NASA’s Lunar Prospector satellite to count the number of neutrons at various orbital heights. From this, they calculated the neutron lifetime to be 887 seconds.

So the decay time may not be exactly 888 seconds but the beam method returns this result. 

LUCK

What are some other contexts in which these triple digits occur? The Wikipedia article on the topic says that:

In Chinese numerology, 888 usually means triple fortune, as s form a strengthening of the digit 8. On its own, the number 8 is often associated with great fortune, wealth and spiritual enlightenment. Hence, 888 is considered triple. For this reason, addresses and phone numbers containing the digit sequence 888 are considered particularly lucky, and may command a premium because of it.

Several online gambling sites incorporate the number:

• 888casino: formerly Casino-on-Net, is an online casino founded in 1997 and based in Gibraltar. It is one of the Internet's oldest casinos, and in 2013 it became the first exclusively online casino to be licensed in the United States.
  
  
• 888 Holdings PLC, (LSE: 888) known commonly as 888.com, is a public company which owns several popular gambling brands and websites. 888 is based in Gibraltar. It is listed on the London Stock Exchange and is a constituent of the FTSE 250 Index. The business was founded in May 1997 by Israeli entrepreneurs Avi and Aaron Shaked and Shay and Ron Ben-Yitzhak, two sets of brothers, as Virtual Holdings Limited. 
  
  
• 888poker, formerly Pacific Poker, is an international online poker card room and network owned by 888 Holdings. 888poker was established in 2002, and is based in Gibraltar.
  
  
• 888sport (pronounced as "Triple Eight Sport") is a multinational online sports gambling company headquartered in Gibraltar. It was founded in 2008 and is a subsidiary of 888 Holdings plc. The company provides online sports betting, predominantly in European markets.

MATHEMATICS

• There is an 888math.com website that offers tutoring at $8.88 per hour.
  
  
  
  
  
• Mathematically, \(888^3 = 700227072\) is the smallest cube in which each digit occurs exactly three times. The list up to one million of such numbers is (permalink):

888, 56592, 58524, 65577, 70869, 78183, 496941, 512427, 516267, 517461, 557949, 565920, 581421, 585558, 661959, 711828, 713772, 723627, 724983, 733053, 739563, 764472, 781830, 877242, 988458

• It is the only cube in which three digits occur three times. For example, the next number in the previous sequence (56592) has a cube of 181244621426688 but there are four digits that occur three times.
  
  
• 888 the smallest multiple of 24 whose digit sum is 24 and, as well as being divisible by its digit sum, it is divisible by all of its digits.
  
  
• 888 and 24 show up again in the former's membership of OEIS 236661 where 888 counts the number of partitions of 24 that have a standard deviation greater than 2. Permalink.
  
  
• 888 can be written using four 4's:    \(4!+\dfrac{4! \times 4!}{\sqrt{.4}}\)
  
• Other properties of the number include its being a happy, Harshad, Moran, nude, strobogrammatic, modest, congruent, amenable, practical, abundant, pseudoperfect and Zumkeller number (see Numbers Aplenty).
  
  
• The 8's are involved in 888 again thanks to its membership of OEIS A127335.
  
  

A127335

Numbers that are the sum of 8 successive primes.

 The sequence runs:

77, 98, 124, 150, 180, 210, 240, 270, 304, 340, 372, 408, 442, 474, 510, 546, 582, 620, 660, 696, 732, 768, 802, 846, 888

The eight successive primes in the case of 888 are 97, 101, 103, 107, 109, 113, 127 and 131 with an average of 111. Both 111 and 888 are of course repdigits along with the infamous 666 or number of the beast.

• 888 arises in the context of aliquot sequences via OEIS A014360:
  
  

A014360

Aliquot sequence starting at 552.

The sequence begins:

552, 888, 1392, 2328, 3552, 6024, 9096, 13704, 20616, 30984, ...

To quote from Wolfram Alpha:

It has not been proven that all aliquot sequences eventually terminate and become periodic. The smallest number whose fate is not known is 276. There are five such sequences less than 1000, namely 276, 552, 564, 660, and 966, sometimes called the "Lehmer five". 

LABOUR

The three 8's are associated with the labour movement as shown in Figure 2:

Figure 2

CHRISTIANITY

The number is strongly associated with Jesus as well via Greek language Gematria:

Figure 3

The close connection between 666 and 888 can be seen in Figure 4 that features the solar magic square. I've written about the magic squares of the Sun, Moon and planets in a previous blog most titled Magic Squares.

Figure 4: source

Figure 5 shows how the two numbers, 666 and 888, are closely linked. In the diagram, the circled numbers on the magic square form the Greek letter “X” which is the “sign” of Christ. The sum of all the numbers in the field of the magic square of the sun (666) added to the sum of the numbers in the twelve Christ circles (222) is equal to the number of Jesus (888).

Figure 5: source

Figure 6 explores the 888 connection with Jesus even further. It should be remembered that the New Testament was written originally in Greek. To quote:

The 24 letters of the Greek alphabet has an 8-8-8 structure. The first row of eight letters represent units, the second row of eight letters represent tens, the third row of eight letters represent hundreds. If you look inside any Greek dictionary you will see this 8-8-8 letter-number table. Every literate Greek person alive during the time of Christ knew it.

The Greek alphabet was invented almost one thousand years before the birth of Jesus. At the time it was invented, every one of the 24 letters in the Greek alphabet represented a number as well as a sound. Since every name and word in the Greek language had a corresponding numerical value, words could be expressed as numbers and numbers could be expressed as words.  This made the Greek alphabet a public cipher and immediately encouraged two numerical practices called Isopsephia and gematria. 

Isopsephia is the practice of converting words and names into numbers. Gematria is the practice of converting numbers into geometry. The number value of "Jesus" is "888." The above circle has a circumference of 888 units, the same value as the 8-8-8 table structure inscribed inside it. Can anyone honestly believe the authors of the gospels did not use this alphabetic cipher tool when they sat down to write? Source.

Figure 6: source

SYNCHRONICITY

Synchronicity struck this morning on my morning walk when I came across the car number plate B 888 AVJ. This is the morning immediately following my post of the previous day. I'd never seen this number plate before, as far as I remember, and I often note car number plates during my walks.

Ultramagic Squares

Here is a definition of an ultramagic square:

A magic square is associative if the sum of any two elements symmetric about its center is the same. A magic square is pandiagonal if the sum of the numbers in any broken diagonal equals the magic constant. A magic square is ultramagic if it is associative and pandiagonal. Ultramagic squares exist for orders n>=5. Source.

Using this as a starting point, let's understand what is meant by a pandiagonal magic square. Here is a definition taken from a most useful website:

Pandiagonal magic squares are magic squares, where also the broken diagonals sum to the magic constant. This means when you go off of one edge on a diagonal, continue (wrap-around) to the corresponding cell on the opposite edge. These squares are considered as one of the top classes of magic squares.

Figures 1 and 2 show clearly what is meant by a "broken diagonal" and show a 5 x 5 magic square that is pandiagonal. 

Figure 1

Figure 2

The magic square in Figures 1 and 2 however, is not associative. Using the central square (24) as a reference point, we note that, up-down 3 + 12 = 15 but left-right 20 + 6 = 26. These must be equal for a magic square to be associative. Figure 3 shows a 5 x 5 magic square that is both pandiagonal and associative, and thus ultramagic.

Figure 3

The magic constant for this square is 65 and it can be seen that all rows, columns, main diagonals and broken diagonals all add to this number. Furthermore, the up-down 6 + 20 = 26 and the left-right 2 + 24 = 26 are this time equal as are all the other symmetric pairs of elements.

Figure 3 shows a 7 x 7 ultramagic square:

Figure 4: source

Figures 5, 6 and 7 show 6 x 6, 7 x 7 and 8 x 8 prime ultramagic squares with magic constants of 990, 4613 and 2040 respectively:

Figure 5: source

Figure 6: source

Figure 8: source

These magic constants (990, 4613 and 2040) are the lowest possible and form part of OEIS A257316:

A257316

Smallest magic constant of ultramagic squares of order \(n\) composed of distinct prime numbers.

The sequence runs 3505, 990, 4613, 2040 with 3505 being the magic constant (not shown) for the 5 x 5 ultramagic square with minimal magic constant. The following bounds for the next terms are known:

• 12249 <=a(9) <=13059
• 4200 <=a(10) <=46150
• a(11) >= 26521
• a(12) >= 8820
• a(13) >= 49439
• a(14) >= 16170
• a(15) >= 74595
• a(16) >= 21840

My attention was attracted to the topic because today I turned 26521 days old and this number happens to be the lower bound for the 11 x 11 prime ultramagic square with minimal magic constant. The exact composition of such a square is presumably still not known.

My earlier posts on Magic Squares are:

• Magic Squares on 21st September 2020
• Prime Semi-Magic Squares on 7th May 2021
• Anti-Magic Squares on 17th July 2018